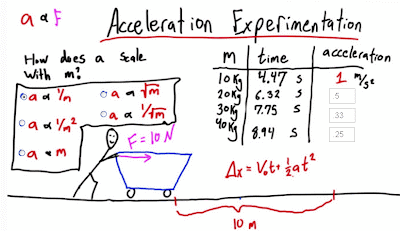
Unit 3 - Lecture 16 ==> Mass & acceleration relationship

Using the data given

• Decomposing  $$ \Delta{x} = V_0 t + \frac{1}{2} a t^2 $$ :
• Since the initial velocity is zero, $$ V_0 t = 0 $$
• Thus we have $$ \Delta{x} = \frac{1}{2} a t^2 $$
• Solving for a, $$a = \frac{2\Delta{x}}{t^2} $$
• Calculate 4 cases for acceleration using force of 10 Newtons

  Mass	Time	Acceleration	Acceleration Rounded
  10 kg	4.47	1.0009559129	1.00
  20 kg	6.32	0.500721038295	0.50
  30 kg	7.75	0.332986472425	0.33
  40 kg	8.94	0.250238978224	0.25

• $$\mbox{Acceleration} \varpropto \mbox{Mass}$$
• So find a relationship where the constant c is a constant
• Try $$ c =  a * m $$
• Calculate 4 cases for the constant c

Mass	Acceleration	Constant c
10	1.00	10
20	0.50	10
30	0.33	10
40	0.25	10

• And no other combination results in a constant constant
• Thus as a goes up or down, m must go down or up the same amount for F to  remain a fixed value.
• Thus F = c * m, and we have just calculated the force of gravity. 

Conclusions

• By observation, acceleration is directly proportional to the force applied.
• And as mass increases, acceleration proportionality decreases.
• $$ a \varpropto \frac{1}{m} $$
• And since $$ F = \mbox{constant} * m $$
• $$ c = 10 $$
• $$ F = 10 * m $$